Segmentize
export segmentize
export LinearSegments, GeodesicSegmentsThis function "segmentizes" or "densifies" a geometry by adding extra vertices to the geometry so that no segment is longer than a given distance. This is useful for plotting geometries with a limited number of vertices, or for ensuring that a geometry is not too "coarse" for a given application.
Info
We plan to add interpolated segmentization from DataInterpolations.jl in the future, which will be available to any vector of point-like objects.
For now, this function only works on 2D geometries. We will also support 3D geometries, as well as measure interpolation, in the future.Examples
import GeometryOps as GO, GeoInterface as GI
rectangle = GI.Wrappers.Polygon([[(0.0, 50.0), (7.071, 57.07), (0, 64.14), (-7.07, 57.07), (0.0, 50.0)]])
linear = GO.segmentize(rectangle; max_distance = 5)
collect(GI.getpoint(linear))9-element Vector{Tuple{Float64, Float64}}:
(0.0, 50.0)
(3.5355, 53.535)
(7.071, 57.07)
(3.5355, 60.605000000000004)
(0.0, 64.14)
(-3.535, 60.605000000000004)
(-7.07, 57.07)
(-3.535, 53.535)
(0.0, 50.0)You can see that this geometry was segmentized correctly, and now has 8 vertices where it previously had only 4.
Now, we'll also segmentize this using the geodesic method, which is more accurate for lat/lon coordinates.
using Proj # required to activate the `Geodesic` method!
geodesic = GO.segmentize(GO.Geodesic(#=ellipsoid params here=#), rectangle; max_distance = 1000)
length(GI.getpoint(geodesic) |> collect)3585This has a lot of points! It's important to keep in mind that the max_distance is in meters, so this is a very fine-grained segmentation.
Now, let's see what they look like! To make this fair, we'll use approximately the same number of points for both.
using CairoMakie
linear = GO.segmentize(rectangle; max_distance = 0.01)
geodesic = GO.segmentize(GO.Geodesic(), rectangle; max_distance = 1000)
f, a, p = poly(collect(GI.getpoint(linear)); label = "Linear", axis = (; aspect = DataAspect()))
p2 = poly!(collect(GI.getpoint(geodesic)); label = "Geodesic")
axislegend(a; position = :lt)
f
There are two methods available for segmentizing geometries at the moment, and you can invoke them by passing the relevant Manifold:
GeometryOpsCore.Planar Type
Planar()A planar manifold refers to the 2D Euclidean plane.
Z coordinates may be accepted but will not influence geometry calculations, which are done purely on 2D geometry. This is the standard "2.5D" model used by e.g. GEOS.
sourceGeometryOpsCore.Geodesic Type
Geodesic(; semimajor_axis, inv_flattening)A geodesic manifold means that the geometry is on a 3-dimensional ellipsoid, parameterized by semimajor_axis (inv_flattening (
Usually, this is only relevant for area and segmentization calculations. It becomes more relevant as one grows closer to the poles (or equator).
sourceBenchmark
We benchmark our method against LibGEOS's GEOSDensify method, which is a similar method for densifying geometries.
using BenchmarkTools: BenchmarkGroup
using Chairmarks: @be
using Main: plot_trials
using CairoMakie
import GeometryOps as GO, GeoInterface as GI, LibGEOS as LG
segmentize_suite = BenchmarkGroup(["title:Segmentize", "subtitle:Segmentize a rectangle"])
rectangle = GI.Wrappers.Polygon([[(0.0, 50.0), (7.071, 57.07), (0.0, 64.14), (-7.07, 57.07), (0.0, 50.0)]])
lg_rectangle = GI.convert(LG, rectangle)POLYGON ((0 50, 7.071 57.07, 0 64.14, -7.07 57.07, 0 50))# These are initial distances, which yield similar numbers of points
# in the final geometry.
init_lin = 0.01
init_geo = 900
# LibGEOS.jl doesn't offer this function, so we just wrap it ourselves!
function densify(obj::LG.Geometry, tol::Real, context::LG.GEOSContext = LG.get_context(obj))
result = LG.GEOSDensify_r(context, obj, tol)
if result == C_NULL
error("LibGEOS: Error in GEOSDensify")
end
LG.geomFromGEOS(result, context)
end
# now, we get to the actual benchmarking:
for scalefactor in exp10.(LinRange(log10(0.1), log10(10), 5))
lin_dist = init_lin * scalefactor
geo_dist = init_geo * scalefactor
npoints_linear = GI.npoint(GO.segmentize(rectangle; max_distance = lin_dist))
npoints_geodesic = GI.npoint(GO.segmentize(GO.Geodesic(), rectangle; max_distance = geo_dist))
npoints_libgeos = GI.npoint(densify(lg_rectangle, lin_dist))
segmentize_suite["Linear"][npoints_linear] = @be GO.segmentize($(GO.Planar()), $rectangle; max_distance = $lin_dist) seconds=1
segmentize_suite["Geodesic"][npoints_geodesic] = @be GO.segmentize($(GO.Geodesic()), $rectangle; max_distance = $geo_dist) seconds=1
segmentize_suite["LibGEOS"][npoints_libgeos] = @be densify($lg_rectangle, $lin_dist) seconds=1
end
plot_trials(segmentize_suite)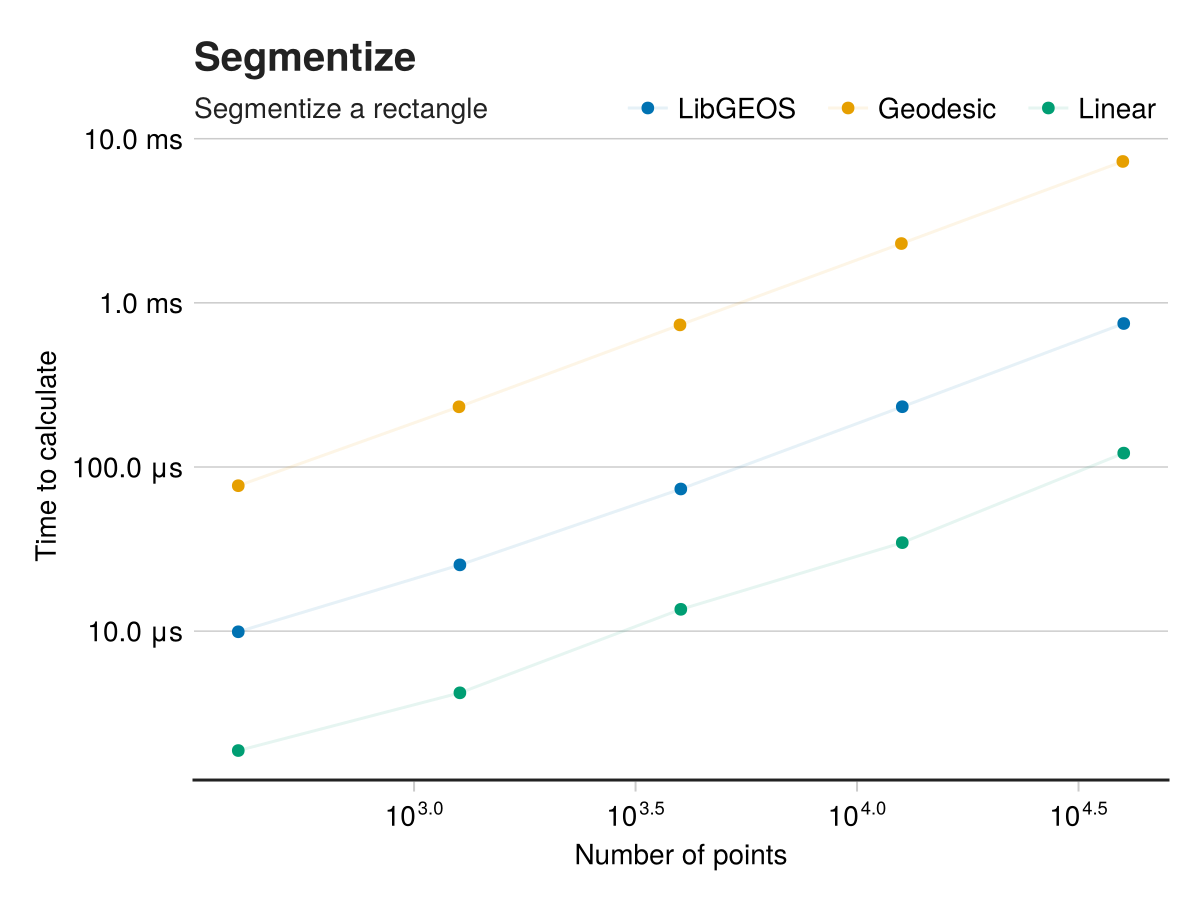
abstract type SegmentizeMethod end
"""
LinearSegments(; max_distance::Real)
!!! warning
This is deprecated - call `segmentize(Planar(), geom; max_distance)` instead.
A method for segmentizing geometries by adding extra vertices to the geometry so that no segment is longer than a given distance.
Here, `max_distance` is a purely nondimensional quantity and will apply in the input space. This is to say, that if the polygon is
provided in lat/lon coordinates then the `max_distance` will be in degrees of arc. If the polygon is provided in meters, then the
`max_distance` will be in meters.
"""
Base.@kwdef struct LinearSegments <: SegmentizeMethod
max_distance::Float64
end
"""
GeodesicSegments(; max_distance::Real, equatorial_radius::Real=6378137, flattening::Real=1/298.257223563)
!!! warning
This is deprecated - call `segmentize(Geodesic(; semimajor_axis, inv_flattening), geom; max_distance)` instead.
A method for segmentizing geometries by adding extra vertices to the geometry so that no segment is longer than a given distance.
This method calculates the distance between points on the geodesic, and assumes input in lat/long coordinates.
!!! warning
Any input geometries must be in lon/lat coordinates! If not, the method may fail or error.
# Arguments
- `max_distance::Real`: The maximum distance, **in meters**, between vertices in the geometry.
- `equatorial_radius::Real=6378137`: The equatorial radius of the Earth, in meters. Passed to `Proj.geod_geodesic`.
- `flattening::Real=1/298.257223563`: The flattening of the Earth, which is the ratio of the difference between the equatorial and polar radii to the equatorial radius. Passed to `Proj.geod_geodesic`.
One can also omit the `equatorial_radius` and `flattening` keyword arguments, and pass a `geodesic` object directly to the eponymous keyword.
This method uses the Proj/GeographicLib API for geodesic calculations.
"""
struct GeodesicSegments{T} <: SegmentizeMethod
geodesic::T# ::Proj.geod_geodesic
max_distance::Float64
endAdd an error hint for GeodesicSegments if Proj is not loaded!
function _geodesic_segments_error_hinter(io, exc, argtypes, kwargs)
if isnothing(Base.get_extension(GeometryOps, :GeometryOpsProjExt)) && exc.f == GeodesicSegments
print(io, "\n\nThe `Geodesic` method requires the Proj.jl package to be explicitly loaded.\n")
print(io, "You can do this by simply typing ")
printstyled(io, "using Proj"; color = :cyan, bold = true)
println(io, " in your REPL, \nor otherwise loading Proj.jl via using or import.")
end
endImplementation
"""
segmentize([method = Planar()], geom; max_distance::Real, threaded)
Segmentize a geometry by adding extra vertices to the geometry so that no segment is longer than a given distance.
This is useful for plotting geometries with a limited number of vertices, or for ensuring that a geometry is not too "coarse" for a given application.
# Arguments
- `method::Manifold = Planar()`: The method to use for segmentizing the geometry. At the moment, only `Planar` (assumes a flat plane) and `Geodesic` (assumes geometry on the ellipsoidal Earth and uses Vincenty's formulae) are available.
- `geom`: The geometry to segmentize. Must be a `LineString`, `LinearRing`, `Polygon`, `MultiPolygon`, or `GeometryCollection`, or some vector or table of those.
- `max_distance::Real`: The maximum distance between vertices in the geometry. **Beware: for `Planar`, this is in the units of the geometry, but for `Geodesic` and `Spherical` it's in units of the radius of the sphere.**
Returns a geometry of similar type to the input geometry, but resampled.
"""
function segmentize(geom; max_distance, threaded::Union{Bool, BoolsAsTypes} = False())
return segmentize(Planar(), geom; max_distance, threaded = booltype(threaded))
endallow three-arg method as well, just in case
segmentize(geom, max_distance::Real; threaded = False()) = segmentize(Planar(), geom, max_distance; threaded)
segmentize(method::Manifold, geom, max_distance::Real; threaded = False()) = segmentize(Planar(), geom; max_distance, threaded)generic implementation
function segmentize(method::Manifold, geom; max_distance, threaded::Union{Bool, BoolsAsTypes} = False())
if max_distance <= 0
throw(ArgumentError("`max_distance` should be positive and nonzero! Found $(max_distance)."))
end
_segmentize_function(geom) = _segmentize(method, geom, GI.trait(geom); max_distance)
return apply(_segmentize_function, TraitTarget(GI.LinearRingTrait(), GI.LineStringTrait()), geom; threaded)
end
function segmentize(method::SegmentizeMethod, geom; threaded::Union{Bool, BoolsAsTypes} = False())
@warn "`segmentize(method::$(typeof(method)), geom) is deprecated; use `segmentize($(method isa LinearSegments ? "Planar()" : "Geodesic()"), geom; max_distance, threaded) instead!" maxlog=3
new_method = method isa LinearSegments ? Planar() : Geodesic()
segmentize(new_method, geom; max_distance = method.max_distance, threaded)
end
_segmentize(method, geom) = _segmentize(method, geom, GI.trait(geom))
#=
This is a method which performs the common functionality for both linear and geodesic algorithms,
and calls out to the "kernel" function which we've defined per linesegment.
=#
function _segmentize(method::Union{Planar, Spherical}, geom, T::Union{GI.LineStringTrait, GI.LinearRingTrait}; max_distance)
first_coord = GI.getpoint(geom, 1)
x1, y1 = GI.x(first_coord), GI.y(first_coord)
new_coords = NTuple{2, Float64}[]
sizehint!(new_coords, GI.npoint(geom))
push!(new_coords, (x1, y1))
for coord in Iterators.drop(GI.getpoint(geom), 1)
x2, y2 = GI.x(coord), GI.y(coord)
_fill_linear_kernel!(method, new_coords, x1, y1, x2, y2; max_distance)
x1, y1 = x2, y2
end
return rebuild(geom, new_coords)
end
function _fill_linear_kernel!(::Planar, new_coords::Vector, x1, y1, x2, y2; max_distance)
dx, dy = x2 - x1, y2 - y1
distance = hypot(dx, dy) # this is a more stable way to compute the Euclidean distance
if distance > max_distance
n_segments = ceil(Int, distance / max_distance)
for i in 1:(n_segments - 1)
t = i / n_segments
push!(new_coords, (x1 + t * dx, y1 + t * dy))
end
endEnd the line with the original coordinate, to avoid any multiplication errors.
push!(new_coords, (x2, y2))
return nothing
endNote
The
_fill_linear_kerneldefinition forGeodesicSegmentsis in theGeometryOpsProjExtextension module, in thesegmentize.jlfile.
This page was generated using Literate.jl.